Authors:
(1) Dorian W. P. Amaral, Department of Physics and Astronomy, Rice University and These authors contributed approximately equally to this work;
(2) Mudit Jain, Department of Physics and Astronomy, Rice University, Theoretical Particle Physics and Cosmology, King’s College London and These authors contributed approximately equally to this work;
(3) Mustafa A. Amin, Department of Physics and Astronomy, Rice University;
(4) Christopher Tunnell, Department of Physics and Astronomy, Rice University.
Table of Links
2 Calculating the Stochastic Wave Vector Dark Matter Signal
3 Statistical Analysis and 3.1 Signal Likelihood
4 Application to Accelerometer Studies
4.1 Recasting Generalised Limits onto B − L Dark Matter
6 Conclusions, Acknowledgments, and References
A Equipartition between Longitudinal and Transverse Modes
B Derivation of Marginal Likelihood with Stochastic Field Amplitude
D The Case of the Gradient of a Scalar
2 Calculating the Stochastic Wave Vector Dark Matter Signal
2.1 The Dark Photon Field

With non-linear gravitational clustering, we expect an equipartition of longitudinal and transverse helicities of the plane waves in our vicinity, regardless of whether the early universe production mechanism favors one over the other.

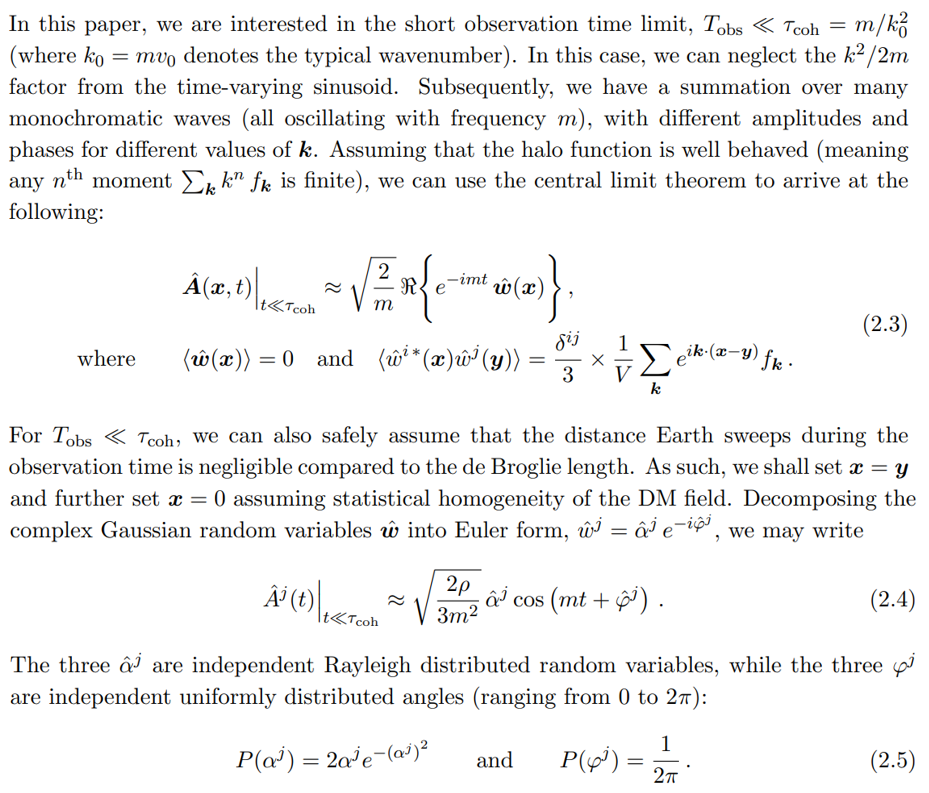
The three components become statistically independent, mimicking three independent scalars.[3] Also note that our result is similar to the case of the gradient of a scalar [45], in the sense that there are 6 independent normal random variables to describe the DM field at a given location and short time scales.
2.1.1 Equipartion and Ellipses
In this subsection, we further justify our assumption of equipartition in the previous section.
Equipartion and Vector Field Ellipses: There exist various production mechanisms where disparate amounts of longitudinal (spin-0) and transverse (spin-1) helicities are produced [20, 21, 23, 25]. That is, for every k, there could be different amounts of longitudinal and transverse components of Ψk [4]. However, owing to non-linear gravitational clustering, such disparity within the two sectors is expected to have disappeared by now within our local cosmic vicinity. With non-linear gravitational clustering, we expect virialization, leading to the equipartition of energy within all three degrees of freedom (dof). This would result in 2/3 of the total power being contained within the (two) transverse dof and the remaining 1/3 within the longitudinal ones. In this case, the vector field at each point (within a coherence region), which is formed out of a sum over a large number of Fourier modes, roughly traces out a randomly oriented ellipse (as opposed to oscillating along some fixed direction). This can be seen by noting that within time scales and length scales much smaller than the coherent ones, the spin current [31, 64] is negligible since it scales with the typical speed σ. Hence, the local spin density (given by s = A × A˙) is conserved, implying that the local field vector A can execute a two-dimensional sinusoidal motion in general, i.e. it sweeps an ellipse. See Fig. 1 for a visualization and a description of this evolution. Such elliptical motion is appropriately used in, for example, [65–67]
In Appendix A, we discuss equipartition in greater detail, providing evidence for it via concrete halo-formation 3D simulations of the Schrodinger field Ψ.
Random Linear Polarization: Some studies make the assumption of linear polarization; i.e. the vector field oscillates along a line in a fixed direction with this direction changing randomly every coherence time (see for example [40, 68–70]). However, as we argued above based on equipartition, an elliptical motion of the vector field is what one should expect. Nevertheless, we note that a preference for linear (circular) polarization could be generated by allowing for a non-gravitational attractive (repulsive) self-interaction [71– 73]. While these works argue for such a preference in isolated soliton-like configurations, whether a significant preference for linear (circular) polarization within each coherence patch can be achieved dynamically remains to be seen.
Fixed Direction: There could exist a misalignment production mechanism for vectors where the entire observable Universe (or at least a large portion of it) has the vector field oscillating in a fixed direction [74, 75]. Such setups indeed lead to a fixed direction of oscillation of the vector field (apart from randomly oriented small perturbations). This would be distinct from the equipartition case we consider. However, there are difficulties associated with their production mechanisms [76–78].
This paper is available on arxiv under CC BY 4.0 DEED license.